Derniers numéros
I | N° 1 | 2021
I | N° 2 | 2021
II | N° 3 | 2022
II | Nº 4 | 2022
III | Nº 5 | 2023
III | Nº 6 | 2023
IV | Nº 7 | 2024
IV | Nº 8 | 2024
V | Nº 9 | 2025
> Tous les numéros
Dossier : Du rythme, entre schématisation et interaction
|
From Continuity to Rhythm Juan Felipe Miranda Medina Publié en ligne le 30 juin 2022
|
|
|
This work addresses rhythm as a continuous becoming, and as that which forms and integrates unities. In order to do so, it focuses on the relationship between rhythm and continuity, departing from the opposition between continuity and discontinuity as set out by Greimas and taken over by E. Landowski. This opposition is in turn analysed in terms of three other oppositions : it is translated into the opposition of self-identity vs difference (section 2), of unity vs diversity (section 3) and of determinism vs randomness (section 4), which leads to the Leibnizian concept of Harmony. The nature of the difference between Rhythm and Harmony as semiotic concepts will be explained in the conclusions. |
* The author would like to acknowledge Dr. Mari Romarheim Haugen for sharing her insights on the experience of musical rhythm. This work is also indebted to Prof. Cummins for his definition of rhythm in terms of “entrainment”. |
|
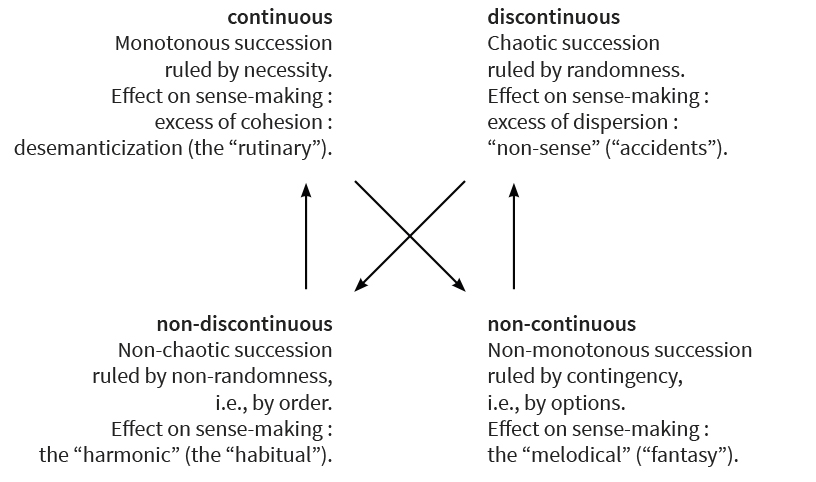
1. Continuity and discontinuity Starting from Greimas’s essay, De l’Imperfection1, Eric Landowski, in Passions sans nom2, reflects on the opposition between continuity and discontinuity and proposes the following schematisation :
|
1 De l’Imperfection, Périgueux, Fanlac, 1987. Spanish transl. R. Dorra, De la Imperfección, México, Fondo de Cultura Económica, 1990. 2 Passions sans nom, Paris, Presses Universitaires de France, 2004. Spanish transl. D. Blanco, Pasiones sin nombre, Lima, Fondo editorial Universidad de Lima, 2018. |
|
Fig. 1. The category continuous vs discontinuous as proposed by E. Landowski.3 In the highest vertex to the right we have continuity, or the continuous, understood as a monotone succession ruled or “programmed” by necessity. Its effect of meaning is an excess of cohesion, which corresponds to a desemanticised world, one that is absolutely identical to itself in every iteration of its succession. Its opposite is the discontinuous, a chaotic succession ruled by chance, whose effect of meaning is dispersion in the form of “non-sense”, a radical alterity that cannot be made sense of. The negation of continuity — the non-continuous — implies negating pure regularity and perfect programming. The author interprets this as allowing for the appearance of a certain “fantasy”, a certain unpredictability in the realisation of action processes, for instance with the introduction of qualitative variables or quantitative modulations along the action process taking place4. |
3 Pasiones sin nombre, op. cit., p. 64 (our translation). 4 By “action process” I mean the goal-oriented doing of a subject to whom the action is attributed. It is analogous to the Greimasian notion of narrative program, where an action or utterance of doing realises a transformation leading from one state to another. In turn, “states” designate relations of conjunction or disjunction between the subject of state and the objects of their quest. |
|
The negation of discontinuity — the non-discontinuous — is then taken to mean the overcoming of randomness and pure chaos. Here we have the possibility of action that is oriented towards producing chains and links between experiences and perceptions that goes together with a “temporal thickness” between people and things that makes it possible to organise the search for signification. This is in stark contrast with the passivity of awaiting revelation (the manifestation of chance in the discontinuous). In the non-discontinuous there is a certain cohesion between different magnitudes : between one action and another, or between an action and its outcome. This articulation and ordering as “putting together” allows for the copresence of subjects, for a world where due to our aesthesic capacity we are affected by feelings. There is here an intriguing question. Landowski associates the non-continuous with fantasy and hence with melody. But what would be the relation between breaking away from perfect regularity and the notion of melody ? Further on, the non-discontinuous appears as linked with harmony. What sort of harmony is being made reference of ? Is this to be interpreted metaphorically, or is it of conceptual importance to the notion of non-discontinuous ? The upcoming sections pursue these questions by translating the opposition continuous vs discontinuous into a series of other oppositions, which will eventually lead us to the relation between continuity, Harmony and Rhythm5. |
5 Capital letters for a word are used to denote a technical (semiotic or philosophical) concept, such as Rhythm, as opposed to musical rhythm. |
|
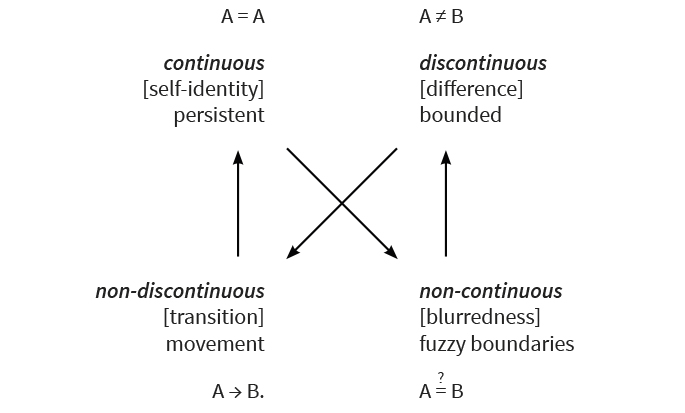
In the above square of continuity vs discontinuity (fig. 1), the former corresponds to absolute regularity (a succession that repeats itself invariably) and the latter to randomness. The opposition between the two, however, is rather ambiguous, as well as the way how randomness is to be construed. Let us approach the opposition continuity vs discontinuity in terms of the opposition of self-identity vs difference instead. This will lead us to a better understanding of the nature of continuity itself. The corresponding semiotic square is shown in Figure 2.
Fig. 2. The category continuous vs discontinuous This square should be approached in a dialectical, iterative manner ; that is, each vertex is never fully independent of the other, but rather an abstraction that, as such, serves as a departure point to understand better the covert aspects of the opposition. We start with the idea that continuity refers to self-identity, to a persistence across a relevant variable, for example, time. Conversely, discontinuity would not refer to identity but rather to difference. Continuity as self-identity asserts “A is A”, while discontinuity as difference asserts “A and B are different”. Yet, difference itself needs a more precise definition. |
|
|
Two things are different if there is a boundary between them that separates them from one another. If one grabs a piece of paper and draws a circle, there is only one boundary, but the paper is split into two : the region of the paper that falls inside the circle and the one that falls outside. In a similar manner, there is a boundary between the pencil that lies on this desk and the desk itself ; both the pencil and the desk are bounded. In the case of a song, the verse and the chorus are bounded in time, that is why we can recognise them as different units that, together with other units such as the introduction, constitute the song6. In other words, the property of being bounded is introduced by the concept of difference. It is in virtue of this “boundedness” that we can conceive of something being composed of distinct parts or units. Units can be arranged across time, space or any other abstract variable thus giving rise to sequences or arrangements of units (e.g., musical notes arranged in time, or mathematical symbols arranged in matrices). Boundaries allow us to associate units (those that fall inside the boundary) and to separate them from other units (those that fall outside the boundary). By association and separation units can be grouped to form new, more complex units. For example, in written language, letters function as elementary units ; groups of letters form words, groups of words form sentences, and so on. Simply put, difference, operating through boundaries, founds hierarchy within a semiotic system. |
6 In music, cadences serve the purpose of bounding or demarcating sequences. More precisely, a musical cadence refers to “the ending of a phrase (...) ; in a larger sense, a cadence may be a demarcation of a half-phrase, of a section of music, or of an entire movement”. M. DeVoto, “Cadence”, Encyclopedia Britannica. |
|
Continuing with the examination of the square in Figure 2, the negation of continuity yields blurredness, which is to say that we cannot perceive or identify the boundaries between A and B : it is uncertain whether A and B are different or not. Blurredness presupposes difference, since as we move from blurredness to difference, the blurredness of the boundaries decreases, i.e., the boundaries become clear. On the other hand, the non-discontinous as non-different corresponds to a fluid transition or transformation from A to B. This vertex is most interesting at a semiotic and philosophical level in relation to continuity. In the non-different, A and B are acknowledged as being different, yet no boundary can be placed at any specific place to delimit where A finishes and where B starts. This, however, should not to be confused with a blurredness in the boundary between A and B. The non-discontinuous as non-different, I claim, defines continuity as such. The continuous is not identical repetition, which is a composite of self-identity and periodicity. Neither is it, as was first proposed, the self-identical. Instead, we follow Leibniz in saying that the continuous is that which is infinitely divisible7. In other words, in continuity boundaries are virtual. This sets it apart from difference, where boundaries are realised, from blurredness, where boundaries are uncertain, and from self-identity, where there are no boundaries. Recapitulating, we started by the premise that continuity is self-identity. After establishing the notion of boundary, however, the conclusion is that continuity as such ought to correspond to the not-discontinuous, i.e., to the case where there is difference or change, but where boundaries are virtual. Applying these considerations to a temporal dimension, and more specifically to music, we can think of two different notes, one after the other (difference), or two different sections (e.g. verse and chorus in a song). We can also think about sections where it is not clear whether the music is still revolving around the same musical motif or around a new one (bluredness), for example in the development section in the Sonata form. It might also be the case that motif A transitions so smoothly to motif B that it takes time before we notice the change (transition). These temporal relations can also be formalised by means of the concept of conjunction / disjunction in Greimasian semiotics. In the context of temporality, we could say that two units that correspond to different temporal sequences (e.g., introduction I and verse V in a song) are in a relation of disjunction (V ? I), while two contiguous units that belong to the same sequence (e.g., two contiguous lines from the verse section) are in a relation of conjunction (v1 ? v2). Furthermore, upon more careful consideration, we realise that if the verse V and the introduction I belong to the same song, the relation of disjunction that marks them as different sections is contained within a relation of conjunction that integrates them within the framework of belonging to the same song. The same applies to the conjunction between v1 and v2, which is subsumed within another conjunction in virtue of the fact that both belong to the same song. This nesting of functions of conjunction and disjunction is what allows us to construct hierarchies within a song, a set of songs, a musical genre, or within any other kind of semiotic object we wish to study. The formation of hierarchies is a necessary condition for a practice to have a syntax8. In thinking about continuity and discontinuity in terms of difference, and resorting to the notion of boundary, we have managed to provide a framework for the formation of new unities and hierarchies. |
7 See R. Arthur, The Labyrinth of the Continuum. Writings on the Continuum Problem, 1672-1686, New Haven, Yale U.P., 2001. G.W. Leibniz, Monadología (1714), Buenos Aires, Orbis, 1983. Since very early in Western philosophy, the problem of continuity has been linked to movement. A video clip consists of a sequence of pictures played in succession at a fixed time rate. For Zeno, however, a video would only provide an illusion of movement. His paradoxes challenge the view that movement can be conceived in terms of a sequence of distinct, successive instants or states, where each state has a clear boundary that separates it from the previous and from the next. Cf. N. Strobach, “Zeno’s Paradoxes”, in A Companion to the Philosophy of Time, West Sussex, John Wiley & Sons, 2013. Merleau-Ponty’s contrasting claim that movement is always in-between the past and the present instant, between the present and the future one, is compatible with Leibniz’s notion of infinite divisibility. See M. Merleau-Ponty and B. Smyth, The Sensible World and the World of Expression. Course Notes from the Collège de France, 1953, Evanston, Northwestern U.P., 2020. 8 A.J. Greimas and J. Courtés, Semiótica. Diccionario razonado de la teoría del lenguaje, Madrid, Gredos, 1982, p. 383. |
|
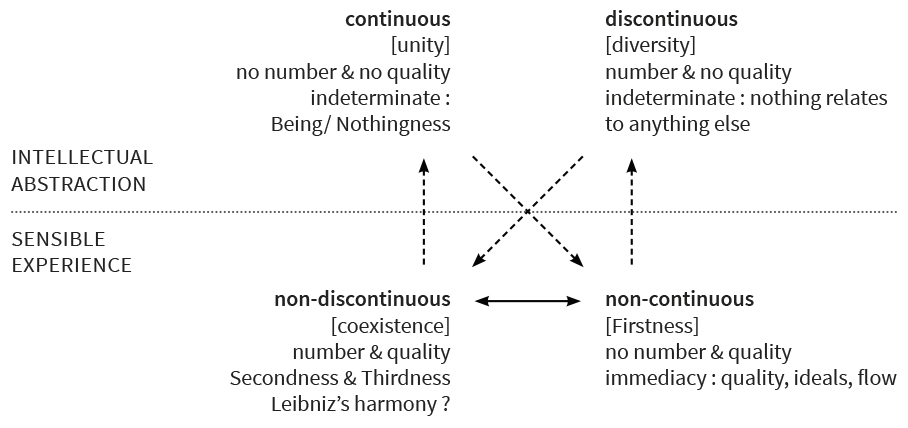
This section interprets the opposition of continuity vs discontinuity in terms of the opposition of unity vs diversity. The purpose is to approach Landowski’s reference to the notions of harmony and coexistence in Section 1. The semiotic square resulting from this new opposition is shown in Figure 3.
|
|
|
Fig. 3. The category continuous vs discontinuous The continuous in this context is construed as a unity in the most abstract sense embraced by several philosophers such as Parmenides or Hegel and his concepts of Being and Nothingness9. What characterises the concept of being as unity (“The One”) is its indeterminacy. It is a category that exists as an intellectual abstraction. It has been understood by some philosophers as that which underlies any particulars (whether sensible or inteligible). Fortunately, we do not need to make any philosophical commitments for the purpose of this semiotic analysis. We translate this indeterminacy proper of unity as that which possesses no number and no quality. Its opposite, diversity, is also an intellectual abstraction where we recognise number (there is more than one single thing) but deny quality. That is to say, in diversity as an abstraction, nothing that is relates to anything else. One might think about diversity colloquially when saying, for example : “This is a diverse country since its inhabitants come from diverse ethnic backgrounds”. This would not correspond to the notion of abstract radical diversity that opposes unity, since difference and diversity in this example are asserted only in virtue of the common category of people that inhabit the same country. The corollary is that diversity as an abstract possesses number but no quality, since any qualitative comparison would already entail a commonality between existents, and if there is something in common, then absolute diversity is no more. |
9 Cf. C.H. Kahn, “The thesis of Parmenides”, The Review of Metaphysics, 1969, pp. 700-724. G.W.F. Hegel, Science of logic (1812), London, Routledge, 2014. |
|
On the other hand, the negation of unity results in Peirce’s category of Firstness. Firstness is an ideal, but it fits well with some of our sensible experiences. For example, taking a walk, one may experience the brightness of the sunlight as a direct quality, without, at that moment, associating it to the sun or to the absence of clouds. Although Peirce’s Firstness is not only aplicable to sensible qualities, it fits the condition of being about quality without being about number since it can be, when experienced, not associated to an existent. The negation of diversity results in coexistence, where there is both number and quality. The multiplicity proper of the sensible world is acknowledged, and quality is asserted in virtue of the fact that the elements or objects of the sensible world are in interaction with each other. These interactions can be based on dyadic and triadic relations. Dyadic relations correspond to relations of association and difference, as discussed in Section 2. As their name suggests they involve two relata, and can therefore be thought of as immediate. Triadic relations involve a third relata as a mediator10. Mediation involves the possibility of grouping A and B as belonging to a common category C. The nesting of conjunctions and disjunctions referred to in the previous section, for example, which allows for a thing to be constituted of parts, is already presupposing mediation. Causality requires mediation, since the relation between cause and event is in turn mediated by a second cause that explains the first one, which is in turn mediated by another cause, and so on. Furthermore, mediation enables relations of equivalence, where, for example, {A1, A2 and A3} are equivalents. They stand as types of token A and can be used interchangeably depending on the context. If the relations of association and difference allow for the formation of sequences and hence of a syntagmatic dimension; the relations of equivalence, substitution and categorisation, enabled by mediation, give rise to the paradigmatic dimension of a system. |
10 Since this work is undertaken from a Greimasean approach, I avoid going very deep into Peirce’s notions of Firstness, Secondness and Thirdness. Notwithstanding, dyadic and triadic relations could be fruitfully approached from Peircean semiotics. |
|
In addition, the coexistence resulting from quality and number is not limited to the realm of sensible experience but can apply to abstract objects as well. In mathematics, for example, coexistence takes place in the form of mathematical spaces. A mathematical space “is a set of mathematical objects with an associated structure”. This structure “can be specified by a number of operations on the objects of the set” that “must satisfy certain general rules” or axioms of the space11. Take the case of integer numbers 11 and 4. These numbers are numerically different since they stand for different numeric values, but they are qualitatively different as well in virtue of their properties, i.e., in virtue of the relations they hold to other numbers. Number 11 is odd and prime, while 4 does not satisfy any of those properties. Number 4 can be written in the form xx where x=2, whereas 11 can not. Coexistence, then, implies a set of objects put into dyadic and triadic relations that bring about change in the state of affairs. Dyadic relations have the opposition self-identity vs difference as their basis and give rise to association, sequencing, grouping. Triadic relations are mediated, and enable operations such as categorisation and equivalence. |
11 M. Dalarsson and N. Dalarsson, Tensors, relativity, and cosmology, London, Academic Press, 2015, p. 13. |
|
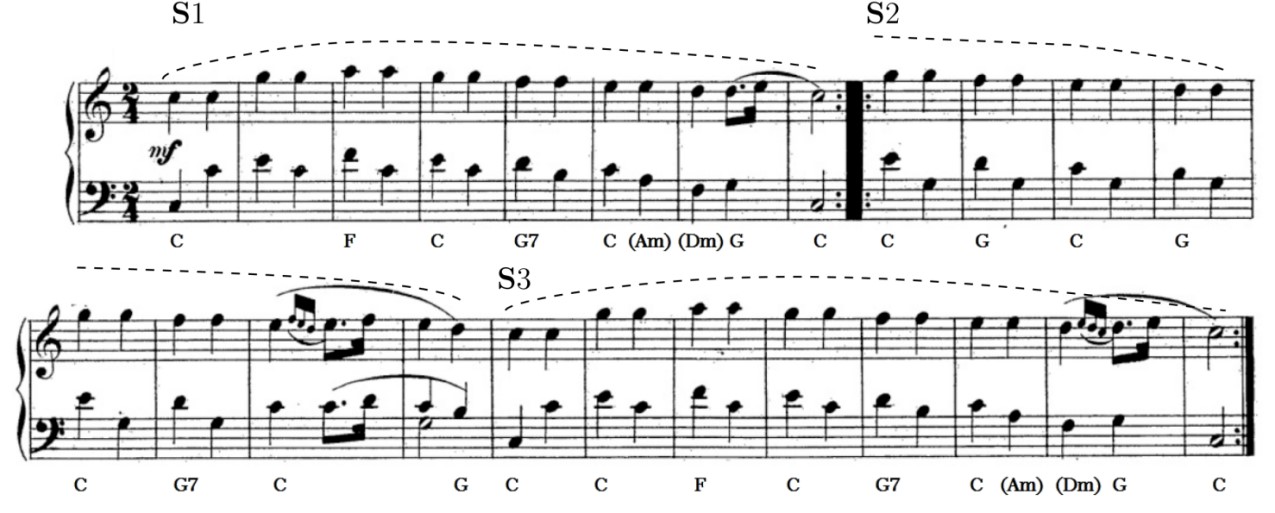
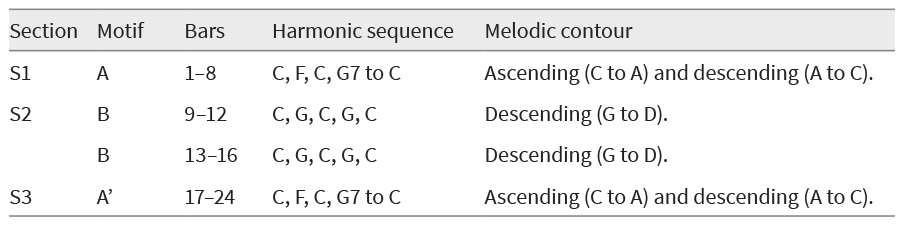
3.1. Coexistence and cohesion, melody and harmony Having characterised coexistence, it is now possible to study how coexistence takes place in music in the form of melody and harmony. After studying both as music concepts, I develop a simple structural analysis of the well known song Twinkle, Twinkle, Little Star that illustrates the relations of association and difference (or conjunction and disjunction) postulated so far, as well as the formation of new units from the combination of smaller units. This example is made use of to elucidate further the notion of cohesion. In music, both melody and harmony as musical categories imply relations of elements to each other. The relation of temporal succession is the one most commonly associated with the melodic. In general, in a melody any note or sound holds paradigmatic relations with the adjacent notes that precede it and succeed it. In other words, the pitch of a given note is constrained by the pitch of the notes in its vicinity. Constraint is not determination, since a constraint based on adjacent notes bounds the options available at the moment of choosing an equivalence for that note, but it does not determine them. Constraint ensures continuity and cohesion within the melody. In most of the popular music genres (e.g., pop, rock, Latin American music, world music) random melodic jumps are regarded as aesthetically unpleasant. If deployed within a genre it will be because other kinds of relations between successive notes will hold. Harmony in Western music theory is usually regarded as the relations that many voices sounding at the same time hold to each other. In an oversimplified fashion, sometimes harmony is thought to be about the “parallel” relations between notes (i.e., notes that sound at the same time). Melody, is associated with “serial” or sequential relationships between notes (i.e., the order in which notes are played). This, however, is a mistake. Harmony is certainly concerned with “parallel” relations addressed in tonal music in terms of chords. Nevertheless, harmony is also fundamentally determined by sequential relations, hence the notion of chord progressions or harmonic circles. There are principles or rules (whether explicit or tacit) that constrain which chord can come before or after any other chord. The relation of sequential dependence that applies to melody applies to harmony as well. In many of the genres that one may enjoy on the radio, YouTube or Spotify, these sequential relations take the abstract form of departing from a consonance, transitioning to a dissonance according to certain rules and then back to a consonance. Even if dissonances add richness and color to the music by means of contrast within the notes the chord comprises, their place in the syntagmatic chain is regulated to fit in the chord progression that composes a song, theme or music piece. In summary, and stated technically, paradigmatic and syntagmatic relations apply both to musical harmony and melody, but harmony adds another dimension that is not present in melody (and in general not even present in spoken language12), that is, coexistence. This is because harmony contemplates the idea of several voices sounding at the same time, coexisting, and regulates their simultaneous interaction, but with a projection in time. These concepts will be better illustrated by means of a practical example. Take the case of the children song Twinkle, Twinkle, Little Star shown in Figure 413. The song is composed of three sections (S1, S2 and S3). The dotted lines indicate the melodic contours. In the very opening of the song there is a jump of a fifth (from C to G) which continues to progress upwards in bar 3 (from G to A), but from bar three to the end of section S1 the melody descends progressively, one note at the time (A, G, F, E, D, C). This melodic contour results from a syntagmatic dimension operating behind. It would have been awkward, for example, if after having reached the A in bar 3, the melody continued to ascend without being compensated by downward motion. It would also had been unpleasant to unexpectedly encounter a note that does not correspond to the tonality of C major, such as D#. The incorporation of this D# would have broken the continuity of section S1, since it would have bared no relation with the notes that come after it either (the descending progression from G to C). Mozart does use chromatisms in his variatons such as G#, but he writes the entire section or motif to make room for these chromatisms (i.e., paradigmatic and syntagmatic relations are taken care of). |
12 Dialogues in spoken language, for example, most often take the form of a temporal alternation between the speakers. It can be that a speaker interrupts another or speaks before the other finished their sentence. This however, is more of an exception than a rule. In the case of Spanish and English, one can safely say that there are no rules about what one can speak while the other is speaking or what one should not say simultaneously to the other. In terms of our ongoing discussion, we could say that “melody” is contemplated and regulated in language, but not harmony. 13 A rendition is accessible at https://youtu.be/xyhxeo6zLAM. |
|
Fig. 4. Score of the song Twinkle, Twinkle, Little Star as it appears in Mozart’s variations.14 |
14 Adapted from W.A. Mozart, Ah ! vous dirai-je, Maman, K. 265 (1781), IMSLP, 2009. |
Harmony as coexistence is present in Twinkle, Twinkle, Little Star in the fact that there are two voices that are related to each other : the theme of the song is on the G-clef while the accompanying voice, subordinated to the theme, is on the left hand. The notes played simultaneously imply a chord. For example, bars 1 and 2 from the chord of C major, while in bar 3 we transition to an F major, in bar 4 to a C, bar 5 to G7 and from then we progress to reach C major again by the end of section S1. First of all, the chord in a given measure is constrained by the tonality of the song (C major), but also by the chords that preceded it and follow it (i.e., there is a syntagmatic dimension). The notion of chord, and the fact, that in this case the melody is given and the accompaniment is written based on this melody, as well as Mozart’s style of writing, constrain the possible notes in the accompaniment. For example, given that the tonality of the piece is C major, and that the first chord is C major as well, the accompaniment could not have started with a G#, for it neither belongs to the tonality of C major, nor would it, together with the C of the melody in bar 1, have formed the C major chord. In short, the melody is associated with a harmony (a sequence of chords corresponds to the sequence of notes of the melody), and both the melody and the sequence of chords constrain the accompaniment. The observations about section S1 apply to S2 as well. In this case there is a melodic progression or contour that descends stepwise from G to D in bars 9 to 12 and this motif is identically repeated in bars 13 to 16. We can thus say that section S1 is composed of a single motif (A), while section S2 consists of the identical repetition of a new motif (BB), which leads back to a motif A’ in section S3 that is almost identical to motif A in S1. It only differs due to an ornamentation right before the end of the song (bar 23). Hence, in terms of motifs, the structure of the piece has the form ABBA’15. A summary of the structural analysis discussed so far is presented in Table 1. |
15 A’ refers to the fact that this motif is a variation of motif A. |
|
Table 1. Structural analysis of Twinkle, Twinkle, Little Star. A closer look into the relation between motifs A and A’ can provide more insight about cohesion. First, there is, in part, a relation of equivalence between both motifs, since structurally they are almost identical, and we have categorised both as being essentially the same motif. Equivalence is also present in the fact that A’ results from modifying the penultimate bar of motif A and substituting some of its notes by an ornament. However, the presence of the ornamentation in A’ distinguishes it from A and makes it more suitable for the finale of the song, to emphasise closure. Thus, A’ is similar but not trivially interchangeable or replaceable by A. In spite of being almost identical in terms of its constituent parts (notes) to A, the motif A’ is unique in regard to A since it occupies a special role in the constitution of the piece. In these reflections, sensible experience is already implied. Hearing something again and again can be tiresome (the monotonous succession that Greimas-Landowski refer to). Thus, as far as we experience and engage with the world it is possible to have an experience that is very different from the ones preceding it, even if it happens in the same situation and even if at an objective level it involves the same components16. |
16 Landowski reminds us of this in his reflections on habits : cf. op. cit., p. 173. Another powerful reflection on the matter of equivalence, substitution and uniqueness comes from Gilles Deleuze who takes an example in a painting by Monet. Each water lilly is not interchangeable by the other, neither are they mere copies of the first. Rather, each flower carries the power of the first to the power of n. See Difference and Repetition, New York, Columbia U.P., 1994, pp. 1, 21. |
|
Pursuing this line of thought, however, leads us to challenge the view that continuity as a regular, monotonous succession is characterised by an excess of cohesion. On the contrary, regularity is just as incohesive as randomness, but in a different sense. In randomness no element holds any relation to any other. In regularity, any element in the sequence is replaceable and undistinguishable from any other. Cohesion stands for the opposite, for relations between elements that are so strong that any substitution of an element affects the rest of the structure. Is the degree of cohesion of a structure to be determined in a purely objective sense (i.e., based on the plane of expression only) ? Certainly not. In a salsa band, the clave can play the same pattern identically again and again, yet it continues to be meaningful in virtue of its coexistence with the other instruments, and with the different motifs, melodies and sections that unfold. This identical repetition of the clave provides us listeners with the experience of having a stable structure upon which other more mobile structures can rely. Cohesion as mobility can also be thought of in the domain of communications. For example, the transistors that are built in a mobile phone are based on having solid structures of materials that are bounded together (low mobility), yet their electrons will be readily in motion when the battery of the phone is turned on. Finally, cohesion can be applied to many different dimensions. For example, in a band, we can say that its orchestration is highly cohesive if removing the electric guitar or changing it for a piano significantly affects the sound that characterises the band. We can also say that there is a cohesion between the chords of their latest hit if substituting one of the chords for a similar one significantly alters the piece. High cohesion is not always desirable in all regards. Lack of cohesion as substitution enables musical variation, whether it is in the accompaniment of a song where the pianist can improvise with some degrees of freedom, or in improvisation based on jazz standards, where the jazz player sees an opportunity to create a new melody that preserves some of the properties of the original one (e.g., its sequence of chords). Cohesion can also come at different levels within the same practice. This superposition of layers with different degrees of mobility or variability can be used to create a cohesive yet diverse whole ? i.e., a harmonious whole, as is made clear in the next section. Going back to the example of the salsa band, while the clave can be played invariably in a section of the song, the congas might be allowed for a higher degree of variation. The brass instruments might have sporadic melodic interventions that are melodically similar, while the singer develops the melody of the song (the highest degree of dispersion considering repetition, but if the melody is carefully composed, its phrases will have high sequential cohesion as well). The reflections on cohesion presented thus far are poetically phrased in the music film Amadeus. When speaking about a composition by Mozart, his colleague Salieri says : “Displace one note and there would be diminishment, displace one phrase and the structure would fall”. |
|
|
3.2. Harmony as a semiotic concept In this section I set out to address Harmony as a semiotic concept drawing from the analysis developed in previous sections17. Given that in music melody and harmony include the paradigmatic and syntagmatic dimensions, but that harmony has an additional level of generality (namely coexistence), melody is henceforth disposed of as materia prima for a concept. But next comes the question : should Harmony simply be defined as identical to coexistence ? To answer this question we can take inspiration from Leibniz’s notion of Harmony. In his early writings Leibniz defined harmony as “similarity in variety, that is, diversity compensated by identity”18. A more mature Leibniz would later on write : Harmony is when many things are reduced to some unity. For where there is no variety, there is no harmony. Conversely, where variety is without order, without proportion, there is no harmony. Hence, it is evident that the greater the variety and the unity in variety, this variety is harmonious to a higher degree.19 Leibniz’s concept of Harmony allows us to better understand the semiotic square of unity vs diversity (fig. 3). For Leibniz any given coexistence is not necessarily harmonious. There is the condition of maximising both diversity and unity, two variables that oppose each other. Unity can be construed as identity, or in a more dynamic fashion, as the force that compensates and unifies the diverse. This understanding of Harmony can be of great relevance for semiotics in the study of socio-political discourses, for often, these discourses dispose or dismantle diversity in their quest for unification. This is exemplified in discourses that proclaim unity at the expense of invisibilising peoples that do not fit into the government’s political agenda, such as African descendants20. Another attempt of imposing unity in Latin America can be seen in discourses speaking of “unity through mestizaje”, promoting the view that we are all equal given that we are all a mix of different ethnic groups, completely disregarding the fact that systemic conditions prevent indigenous peoples from enjoying the same privileges as the ruling class that preaches unity21. Another example of the pertinence of Harmony can be found in how a living tradition can in time be reduced to a canon of fixed rules, or even worse, to a fixed repertoire of songs and dances. Thus is the case of the Afro-Peruvian revival, where certain genres were (re)invented in the late 1950s through a creative process conducted by different groups of black artists22. Due to different socio-political conditions, however, this creative process was interrupted during the 1980s and a fixed repertoire began to consolidate itself as a canon that is today repeated over and over by professional and semiprofessional Afro-Peruvian groups23. Summarising, there is a risk of imposing unity through political actions at the expense of diversity, as there is a risk in proclaiming unity at the expense of invisibilising others, or of arriving at Landowski’s notion of a senseless regularity by giving up diversity altogether. An example of the converse, of pursuing diversity without unity, could be anarchism or extreme individualism, two phenomena that are not strange to us in the capitalist dynamics of today’s world, or, perhaps more idealistically, some form of “admission” of the Other24. Harmony as a semiotic concept is hence defined as coexistence that aims at maximising both diversity and unity. The “aiming at” is important, since Harmony is not a static state of affairs, but rather a dynamic process. First, it is the result of two forces that are continuously at play (the force of unification and the force of diversification). Second, it denotes an active tendency of a system or world towards maximising both. |
17 Note the distinction between “Harmony” as a philosophical-semiotic concept and “harmony” as a musical concept. The author already proposed Harmony as a semiotic concept, in relation to counterpoint and Greimas’s “competence”. Cf. J.F. Miranda Medina, “Competence, Counterpoint and Harmony : A triad of semiotic concepts for the scholarly study of dance”, Signata, 11, 2020. 18 G.W. Leibniz, Confessio philosophi. La profession de foi du philosophe (1672), Y. Belaval (ed.), Paris, Vrin, 1965 ; English tr. The Philosopher’s Confession, Paris, Vrin, 1970, pp. 24-110. 19 Cited in L. Carlin, “On the very concept of harmony in Leibniz”, The Review of Metaphysics, 2000 ; also available at G.W. Leibniz, Sämtliche Schriften und Briefe (1677), Berlin, Akademie Verlag, 1999. 20 Cf. G. Kleidermacher, “Africanos y afrodescendientes en la Argentina : invisibilización, discriminación y racismo”, Rita, 5, 2021 ; D.E. Careaga-Coleman, La ausencia de lo afro en la identidad nacional de México : raza y los mecanismos de la invisibilización de los afrodescendientes en la historia, la cultura popular y la literatura mexicana (Ph.D. thesis), The University of New Mexico, 2015. 21 Cf. J. Morales, “Mestizaje, malicia indígena y viveza en la construcción del carácter nacional”, Revista de estudios Sociales, 1, 1998 ; S.C. Sartorello, “Convivencia y conflicto intercultural : jóvenes universitarios indígenas y mestizos en la Universidad Intercultural de Chiapas”, Revista mexicana de investigación educativa, 21, 2016. 22 Cf. J.F. Miranda Medina, “The Afro-Peruvian Renaissance. The Vertex and the Void”, Risa, Vacío y Libertad, p. 149 ; H. Feldman, Black rhythms of Peru : reviving African musical heritage in the Black Pacific, Middletown, Wesleyan U.P., 2006. 23 This process of aestheticization and commodification of Afro-Peruvian cultural expressions has been studied by J. F. León and H. C. Feldman among others. My own fieldwork with the Afro-Peruvian community (since 2013) gives faith of their views. Afro-Peruvian artists continue to create music and dance, but rather than developing genres such as the festejo, landó and the zamacueca, they do so by recurring to “fusing” these genres with more mainstream music such as salsa and reguetón. 24 Cf. E. Landowski, “Segregación vs admisón”, Presencias del Otro, Lima, Fondo Editorial Universidad de Lima, 2007, pp. 19-25. |
|
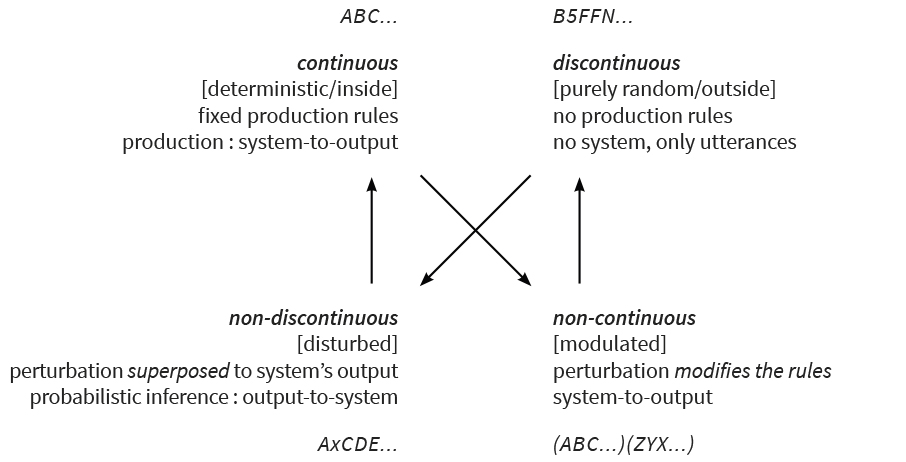
A semiotic concept of Harmony was put forward in the previous section, but thus far Landowski’s interpretation of the discontinuous as randomness has not been addressed. Therefore, a new semiotic square is introduced that translates the opposition continuous vs discontinuous into the opposition deterministic vs purely random (fig. 5). The deterministic regime results in the production of an output (utterances) based on fixed, unalterable production rules. Since we speak of production we have a direction of system-to-output. These are exemplified in the sequence {ABC..}. The purely random, just as pure diversity, is an abstraction, an impossibility. Even with the most powerful computers, algorithms are used to generate pseudo-random sequences of numbers, for how could one generate a sequence of numbers or letters where no element bears any sort of relation to the rest of elements ? In the ideal abstraction of the discontinuous as the purely random there is no system, only an output stream. This idea is exemplified by means of the sequence {B5FFN...}.
Fig. 5. The category continuous vs discontinuous The contradictory of the discontinuous (or purely random) may be viewed as the “disturbed”. It corresponds to an output stream or sequence whose elements bear some relation to each other. By observing the output we can infer (recurring to probability) the functioning of the system or the relation of the elements to each other. This is the chief task of statistics, which takes numbers as its elements. Another way of understanding the probable is thinking that randomness is superposed to the output of the fixed deterministic system that can only produce predictable sequences. This is exemplified in the sequence {AxCDE}, where the “x” instead of the “B” indicates the presence of randomness. The negation of the continuous leads to a crucial case, the modulated. When there is modulation, the behavior or the purely deterministic system is modified, modulated, by a random perturbation. Whereas in the non-discontinuous chance perturbed the output of the system, in the non-continuous it perturbs or modulates the system itself (i.e., its rules of production). This is exemplified with the sequence {(ABC...)} that at some point, due to the presence of randomness, inverts the plus-one progression, yielding the sequence {(ZYX...)}. The opposition of deterministic vs pure randomness is closely related to inside vs outside. That is, the deterministic can only be so under complete enclosure. As soon as the presence of an exteriority becomes latent in the output or in the system itself, pure determinism is no more. Interestingly, this exteriority could also be determined by fixed rules, but due to the fact that it modifies the behavior of the system in question it acquires the statute of being random. For instance, when transmitting the signal from a mobile phone to another mobile phone there is interference due to the walls, to fading and to the multiple trajectories that the signal follows. Even if the laws for each of these “random” phenomena are well established, the receiving end cannot calculate nor predict the causal chain that has distorted the signal, but it can rely on probability to adopt the best strategy to compensate for these limitations. In other words, in semiotics, randomness is relative to a narrative, to an ongoing action process. The former denotes an exteriority in relation to the latter. Perturbations and modulations are thoroughly studied in several disciplines of engineering (e.g., control engineering, signal processing, chemical engineering, computer science). Their systematic analysis will be left for the sequel to this article. For now, we can say that the inside / outside that determinism / randomness configures can be related to the expectations of a perceiving esthesic subject. For example, in the case of music, experimental sound music comes across as pure randomness to listeners that are not familiar with it, even if it is the outcome of a thorough composition process. On the other hand, if we have an identical rhythmic pattern that is repeated without variation there is determinism in that we know exactly what to expect. Determinism can be perturbed the moment a new instrument is superposed to the fixed rhythmic pattern, or when the pattern itself is suddenly varied only once and then continues as before. Modulation, an alteration of the rules of production, could be exemplified by altering the rhythm consistently (as opposed to suddenly), thus yielding a different rhythmic pattern. Modulation can be viewed as the capacity of a competent esthesic subject in that it requires an adjustment of the current state of affairs (rules of production) to a new state of affairs. |
|
|
Conclusions and beginnings : continuity, harmony and rhythm In her writings, Victoria Santa Cruz — a dancer, choreographer, lecturer and one of the most important Afro-Peruvian thinkers — introduces Rhythm as a continuous becoming, as a sort of force with the power to integrate and form new unities25. The nature of continuity and of the integration and formation of new unities have both been the concern of this work. Taking continuity as a pivotal concept, Landowski’s opposition of continuity (a monotonous succession ruled by necessity) vs discontinuity (a chaotic succession ruled by randomness) was explained. So were their contradictories, non-discontinuity as an order which, as the author puts it, has a “harmonic” effect on sense-making, and non-continuity as a non-monotonous succession that is ruled by contingency and that allows for options and for “the melodic”26. Throughout this work I have argued that the original formulation of continuity vs discontinuity to which I refer intermingles too many variables. Hence the need for an analysis in terms of three more specific oppositions : self-identity vs difference, unity vs diversity and determinism vs randomness. |
25 V. Santa Cruz, Ritmo : El Eterno Organizador, Lima, Petróleos del Perú, 2004. 26 Cf. Passions sans nom, op. cit., pp. 50-56. Pasiones sin nombre, op. cit., pp. 63-69. |
|
The study of difference revealed boundary as a key concept capable of grouping elements together into an interiority while leaving others outside, which is analogous to Greimas’s conjunction / disjunction. Conjoining elements together simultaneously disjoins this new group from other elements, which allows for the formation of new units, and is therefore a precondition for hierarchy (e.g., individual notes, motifs, phrases, sections, etc.). Thus, difference corresponds to the realisation of a boundary. Besides, the notion of boundary allows for a different understanding of continuity. The continuous is not the self-identical (which is the closest to monotonous succession), but rather the infinitely divisible. The former knows no boundaries and is hence undeterminate, while boundaries in the latter are virtually present. Infinite divisibility is present in movement as well, i.e., an object that moves is neither only in the present instant nor in the next, and can best be understood as a sense of flow. E.g., in musical rhythm, flow can become manifest in the continuity within a rhythmic motif played repeatedly, while difference as boundedness is at play when we distinguish one repetition from the next one. The study of unity vs diversity led to the concept of coexistence as a plurality of interrelated elements. This is because coexistence is characterised by admitting both number and quality. On the one hand, number entails plurality. On the other hand, quality as the specificity of each element can only arise if the plurality of elements is interrelated (in dyadic or triadic relations). Coexistence in turn implies the paradigmatic and syntagmatic dimensions of semiotic analysis, i.e., the sequencing of elements in a realisation and the possible equivalences between elements in that same realisation. The concept of Harmony was postulated as a specific form of coexistence where there is a tendency to maximise both diversity and unity. From a different perspective, Harmony implies the dynamic interaction between two forces : a unifying force and a diversifying force. Balancing the two is not trivial. For example, some songs may attempt to achieve Harmony at the expense of repeating the same verse with the same beat again and again. Other unfortunate attempts might involve too many musical motifs that are not perceived as related to each other. Harmony is also related to cohesion. A “monotonous succession” is just as uncohesive as a “random succession”, since any element in the succession could be removed from the succession without having an impact on the sequence. The cohesion of an object or system can be studied along several dimensions. For example, in music we can study the cohesion of melodic lines, of the instrumentation of an ensemble, but often the combination of disperse and cohesive layers can yield a greater overall cohesion (one that falls neither into a monotonous succession nor a random one). How does all of this connect to Rhythm as a semiotic concept ? Harmony has been defined as the outcome of the interplay of a force of unification and a force of diversification. Hence, I put forward the thesis that, within a system or object, Rhythm refers to a certain level of Harmony (i.e., unification and diversity are balanced in a certain configuration) with the postulate that entrainment — a notion borrowed from chemical engineering27 — is the unifying mechanism of the system. Naturally, this thesis calls for a continuation of this work in the near future, where entrainment will be defined from a semiotic perspective so that it can be integrated in the development of this theory and related to Landowski’s notion of adjustment. |
27 According to the Collins Dictionary, “entrainment is when a fluid picks up and drags another fluid or a solid” (https://www.collinsdictionary.com/es/diccionario/ingles). More broadly, it may be defined as a “spatiotemporal coordination resulting from rhythmic responsiveness to a perceived rhythmic signal”. Cf. J. Phillips-Silver, C.A. Aktipis and G. Bryant, “The ecology of entrainment : Foundations of coordinated rhythmic movement”, Music perception, 28, 1, 2010, p. 3. |
|
For the present time, the definition of Rhythm I propose relies on all the previous considerations — on boundaries, coexistence, Harmony, cohesion, and, as remains to be shown, on the semiotic regimes of manipulation and adjustment. How does this impact semiotics ? There are a number of research possibilities that this work opens. The semiotic squares resulting from the analysis of continuity can provide a different understanding of Landowski’s four regimes of signification, and perhaps aid in the analysis of these regimes. On the other hand, this work has shown that the concepts of boundary, cohesion and perturbation can have an impact on how we construe semiotic processes. Each of these concepts can be studied in its own right and applied to specific case studies. Finally, the concept of Rhythm carried out in this work is coherent with musical rhythm. Rhythm in music is not simply based on repetition, but rather on the interplay between stable perceived structures (e.g., meter) and more dynamic components such as melodies, rhythmic variations, tonal modulations and different rhythmic sections. In other words, the unifying and diversifying forces are always at play, whether it is in the temporal integration of musical structures into longer sections or in the unification by means of aesthetic conventions that allow the simultaneous sound of several instruments to be enjoyable. At the same time, musical rhythm, when experienced as continuous, drives forward with the power of a continuous flow, that is, of infinite divisibility. |
|
______________ Bibliography Arthur, Richard, The Labyrinth of the Continuum : Writings on the Continuum Problem, 1672-1686, New Haven and London, Yale University Press, 2001. Careaga-Coleman, Dora E., La ausencia de lo afro en la identidad nacional de México : raza y los mecanismos de la invisibilización de los afrodescendientes en la historia, la cultura popular y la literatura mexicana (Ph.D. thesis), The University of New Mexico, 2015. Carlin, Laurence, “On the very concept of harmony in Leibniz”, The Review of Metaphysics, 2000. Dalarsson, Mirjana and Nils, Tensors, relativity, and cosmology, London, Academic Press, 2015. Deleuze, Gilles, Difference and repetition, New York, Columbia University Press, 1994. DeVoto, Mark, “Cadence”, Encyclopedia Britannica, 2017. Feldman, Heidi, Black rhythms of Peru : reviving African musical heritage in the Black Pacific, Middletown, Wesleyan University Press, 2006. Greimas, Algirdas J., De l’Imperfection, Périgueux, Fanlac, 1987 ; transl. R. Dorra, De la Imperfección, México, Fondo de Cultura Económica, 1990. — and Joseph Courtés, Semiótica. Diccionario razonado de la teoría del lenguaje, trad. E. Ballón Aguirre y H. Campodónico Carrión, Madrid, Gredos, 1982. Hegel, Georg W. F., Science of logic (1812), London, Routledge, 2014. Kahn, Charles H., “The thesis of Parmenides”, The Review of Metaphysics, 1969. Kleidermacher, Gisele, “Africanos y afrodescendientes en la Argentina : invisibilización, discriminación y racismo”, Revista Rita, 5, 2021. Landowski, Eric, Presencias del Otro (1997), Lima, Fondo Editorial Universidad de Lima, 2007. — Passions sans nom, Paris, P.U.F., 2004 ; tr. D. Blanco, Pasiones sin nombre. Ensayos de sociosemiótica, Lima, Fondo editorial Universidad de Lima, 2018. — Interacciones arriesgadas (2005), Lima, Fondo editorial Universidad de Lima, 2017. Leibniz, Gottfried Wilhelm, Confessio philosophi. La profession de foi du philosophe (1672), Y. Belaval (ed.), Paris, Vrin, 1965 ; English tr. The Philosopher’s Confession, Paris, Vrin, 1970. — Sämtliche Schriften und Briefe (1677), Berlin, Akademie Verlag, 1999. — Monadología (1714), Buenos Aires, Orbis, 1983. Merleau-Ponty, Maurice and Bryan Smyth, The Sensible World and the World of Expression. Course Notes from the Collège de France, 1953, Evanston, Northwestern University Press, 2020. Miranda Medina, Juan Felipe, “Competence, Counterpoint and Harmony : A triad of semiotic concepts for the scholarly study of dance”, Signata, 11, 2020. — “The Afro-Peruvian Renaissance. The Vertex and the Void”, Risa, Vacío y Libertad, Arequipa, Universidad Nacional de San Agustín de Arequipa, 2022. Morales, Jorge, “Mestizaje, malicia indígena y viveza en la construcción del carácter nacional”, Revista de estudios Sociales, 1, 1998. Phillips-Silver, Jessica, C. Athena Aktipis and Gregory A. Bryant, “The ecology of entrainment. Foundations of coordinated rhythmic movement”, Music perception, 28, 1, 2010. Santa Cruz, Victoria, Ritmo : el eterno organizador, Lima, Petróleos del Perú, 2004. Sartorello, Stefano Claudio, “Convivencia y conflicto intercultural : jóvenes universitarios indígenas y mestizos en la Universidad Intercultural de Chiapas”, Revista mexicana de investigación educativa, 21, 2016. Strobach, Niko, “Zeno’s Paradoxes”, in A Companion to the Philosophy of Time, West Sussex, John Wiley & Sons, 2013. |
|
* The author would like to acknowledge Dr. Mari Romarheim Haugen for sharing her insights on the experience of musical rhythm. This work is also indebted to Prof. Cummins for his definition of rhythm in terms of “entrainment”. 1 De l’Imperfection, Périgueux, Fanlac, 1987. Spanish transl. R. Dorra, De la Imperfección, México, Fondo de Cultura Económica, 1990. 2 Passions sans nom, Paris, Presses Universitaires de France, 2004. Spanish transl. D. Blanco, Pasiones sin nombre, Lima, Fondo editorial Universidad de Lima, 2018. 3 Pasiones sin nombre, op. cit., p. 64 (our translation). 4 By “action process” I mean the goal-oriented doing of a subject to whom the action is attributed. It is analogous to the Greimasian notion of narrative program, where an action or utterance of doing realises a transformation leading from one state to another. In turn, “states” designate relations of conjunction or disjunction between the subject of state and the objects of their quest. 5 Capital letters for a word are used to denote a technical (semiotic or philosophical) concept, such as Rhythm, as opposed to musical rhythm. 6 In music, cadences serve the purpose of bounding or demarcating sequences. More precisely, a musical cadence refers to “the ending of a phrase (...) ; in a larger sense, a cadence may be a demarcation of a half-phrase, of a section of music, or of an entire movement”. M. DeVoto, “Cadence”, Encyclopedia Britannica. 7 See R. Arthur, The Labyrinth of the Continuum. Writings on the Continuum Problem, 1672-1686, New Haven, Yale U.P., 2001. G.W. Leibniz, Monadología (1714), Buenos Aires, Orbis, 1983. Since very early in Western philosophy, the problem of continuity has been linked to movement. A video clip consists of a sequence of pictures played in succession at a fixed time rate. For Zeno, however, a video would only provide an illusion of movement. His paradoxes challenge the view that movement can be conceived in terms of a sequence of distinct, successive instants or states, where each state has a clear boundary that separates it from the previous and from the next. Cf. N. Strobach, “Zeno’s Paradoxes”, in A Companion to the Philosophy of Time, West Sussex, John Wiley & Sons, 2013. Merleau-Ponty’s contrasting claim that movement is always in-between the past and the present instant, between the present and the future one, is compatible with Leibniz’s notion of infinite divisibility. See M. Merleau-Ponty and B. Smyth, The Sensible World and the World of Expression. Course Notes from the Collège de France, 1953, Evanston, Northwestern U.P., 2020. 8 A.J. Greimas and J. Courtés, Semiótica. Diccionario razonado de la teoría del lenguaje, Madrid, Gredos, 1982, p. 383. 9 Cf. C.H. Kahn, “The thesis of Parmenides”, The Review of Metaphysics, 1969, pp. 700-724. G.W.F. Hegel, Science of logic (1812), London, Routledge, 2014. 10 Since this work is undertaken from a Greimasean approach, I avoid going very deep into Peirce’s notions of Firstness, Secondness and Thirdness. Notwithstanding, dyadic and triadic relations could be fruitfully approached from Peircean semiotics. 11 M. Dalarsson and N. Dalarsson, Tensors, relativity, and cosmology, London, Academic Press, 2015, p. 13. 12 Dialogues in spoken language, for example, most often take the form of a temporal alternation between the speakers. It can be that a speaker interrupts another or speaks before the other finished their sentence. This however, is more of an exception than a rule. In the case of Spanish and English, one can safely say that there are no rules about what one can speak while the other is speaking or what one should not say simultaneously to the other. In terms of our ongoing discussion, we could say that “melody” is contemplated and regulated in language, but not harmony. 13 A rendition is accessible at https://youtu.be/xyhxeo6zLAM. 14 Adapted from W.A. Mozart, Ah ! vous dirai-je, Maman, K. 265 (1781), IMSLP, 2009. 15 A’ refers to the fact that this motif is a variation of motif A. 16 Landowski reminds us of this in his reflections on habits : cf. op. cit., p. 173. Another powerful reflection on the matter of equivalence, substitution and uniqueness comes from Gilles Deleuze who takes an example in a painting by Monet. Each water lilly is not interchangeable by the other, neither are they mere copies of the first. Rather, each flower carries the power of the first to the power of n. See Difference and Repetition, New York, Columbia U.P., 1994, pp. 1, 21. 17 Note the distinction between “Harmony” as a philosophical-semiotic concept and “harmony” as a musical concept. The author already proposed Harmony as a semiotic concept, in relation to counterpoint and Greimas’s “competence”. Cf. J.F. Miranda Medina, “Competence, Counterpoint and Harmony : A triad of semiotic concepts for the scholarly study of dance”, Signata, 11, 2020. 18 G.W. Leibniz, Confessio philosophi. La profession de foi du philosophe (1672), Y. Belaval (ed.), Paris, Vrin, 1965 ; English tr. The Philosopher’s Confession, Paris, Vrin, 1970, pp. 24-110. 19 Cited in L. Carlin, “On the very concept of harmony in Leibniz”, The Review of Metaphysics, 2000 ; also available at G.W. Leibniz, Sämtliche Schriften und Briefe (1677), Berlin, Akademie Verlag, 1999. 20 Cf. G. Kleidermacher, “Africanos y afrodescendientes en la Argentina : invisibilización, discriminación y racismo”, Rita, 5, 2021 ; D.E. Careaga-Coleman, La ausencia de lo afro en la identidad nacional de México : raza y los mecanismos de la invisibilización de los afrodescendientes en la historia, la cultura popular y la literatura mexicana (Ph.D. thesis), The University of New Mexico, 2015. 21 Cf. J. Morales, “Mestizaje, malicia indígena y viveza en la construcción del carácter nacional”, Revista de estudios Sociales, 1, 1998 ; S.C. Sartorello, “Convivencia y conflicto intercultural : jóvenes universitarios indígenas y mestizos en la Universidad Intercultural de Chiapas”, Revista mexicana de investigación educativa, 21, 2016. 22 Cf. J.F. Miranda Medina, “The Afro-Peruvian Renaissance. The Vertex and the Void”, Risa, Vacío y Libertad, p. 149 ; H. Feldman, Black rhythms of Peru : reviving African musical heritage in the Black Pacific, Middletown, Wesleyan U.P., 2006. 23 This process of aestheticization and commodification of Afro-Peruvian cultural expressions has been studied by J. F. León and H. C. Feldman among others. My own fieldwork with the Afro-Peruvian community (since 2013) gives faith of their views. Afro-Peruvian artists continue to create music and dance, but rather than developing genres such as the festejo, landó and the zamacueca, they do so by recurring to “fusing” these genres with more mainstream music such as salsa and reguetón. 24 Cf. E. Landowski, “Segregación vs admisón”, Presencias del Otro, Lima, Fondo Editorial Universidad de Lima, 2007, pp. 19-25. 25 V. Santa Cruz, Ritmo : El Eterno Organizador, Lima, Petróleos del Perú, 2004. 26 Cf. Passions sans nom, op. cit., pp. 50-56. Pasiones sin nombre, op. cit., pp. 63-69. 27 According to the Collins Dictionary, “entrainment is when a fluid picks up and drags another fluid or a solid” (https://www.collinsdictionary.com/es/diccionario/ingles). More broadly, it may be defined as a “spatiotemporal coordination resulting from rhythmic responsiveness to a perceived rhythmic signal”. Cf. J. Phillips-Silver, C.A. Aktipis and G. Bryant, “The ecology of entrainment : Foundations of coordinated rhythmic movement”, Music perception, 28, 1, 2010, p. 3. |
|
Résumé : Cette étude prend pour point de départ la catégorie continuité vs discontinuité telle que formulée par E. Landowski. Je soutiens que cette opposition est une structure complexe qui peut avec profit être analysée en termes d’identité vs différence, d’unité vs diversité et de déterminisme vs aléa. La projection de ces oppositions sur le carré sémiotique de Greimas éclaire les notions de différence, de frontière et de cohésion, qui nous servent elles-mêmes de clef pour construire le concept sémiotique d’Harmonie en tant qu’interface entre forces unificatrices et différenciatrices. La conceptualisation de l’harmonie représente selon nous le premier pas pour l’étude du rythme en tant que concept sémiotique. Resumo : A presente investigação parte da oposição entre continuidade e descontinuidade tal como formulada no plano semiótico por E. Landowski. Tal oposição é um complexo que pode ser analisado em termos de identidade vs diferença, unidade vs diversidade e determinismo vs aleatoriedade. Por sua vez, o estudo dessas oposições utilizando o quadrado de Greimas permite uma melhor compreensão dos conceitos de diferença, limite e coesão. Esta tríade será fundamental para a formulação da Harmonia enquanto relação dinâmica entre uma força unificadora e uma força diversificadora. A harmonia, na visão do autor, é o primeiro passo em direção ao estudo do rimo como conceito semiótico. Abstract : This work departs from the opposition between continuity and discontinuity as formulated by semiotician Eric Landowski. I argue that the opposition is a complex that can be fruitfully analysed in terms of self-identity vs difference, unity vs diversity and determinism vs pure randomness. The study of these oppositions deploying Greimas’s square provides an insight into the notions of difference, boundary and cohesion. These will be key to the formulation of a semiotic concept of Harmony as the interplay between a unifying and a diversifying force. Harmony, I claim, is the first step for the study of rhythm as a semiotic concept. Resumen : La presente investigación parte de la oposición entre continuidad y discontinuidad estudiada por el semiota Eric Landowski. Dicha oposición es un complejo que puede ser productivamente analizado en términos de identidad vs diferencia, unidad vs diversidad y determinismo vs aleatoriedad. A su vez, el estudio de estas oposiciones utilizando el cuadrado de Greimas permite una mejor comprensión de los conceptos de diferencia, límite y cohesión. Esta triada será fundamental para la formulación de la Armonía como una relación dinámica entre una fuerza unificadora y una fuerza diversificadora. La Armonía, en la visión del autor, es el primer paso hacia el estudio del ritmo como un concepto semiótico. Mots clefs : continuity vs discontinuity, determinism vs randomness, identity vs difference, rhythm. Auteurs cités : Gilles Deleuze, Algirdas J. Greimas, Eric Landowski, Gottfried W. Leibniz, Maurice Merleau-Ponty, Victoria Santa Cruz Plan : 1. Continuity and discontinuity 1. Coexistence and cohesion, melody and harmony |
|
Pour citer ce document, choisir le format de citation : APA / ABNT / Vancouver |
|
Recebido em 16/10/2021. / Aceito em 13/05/2022. |